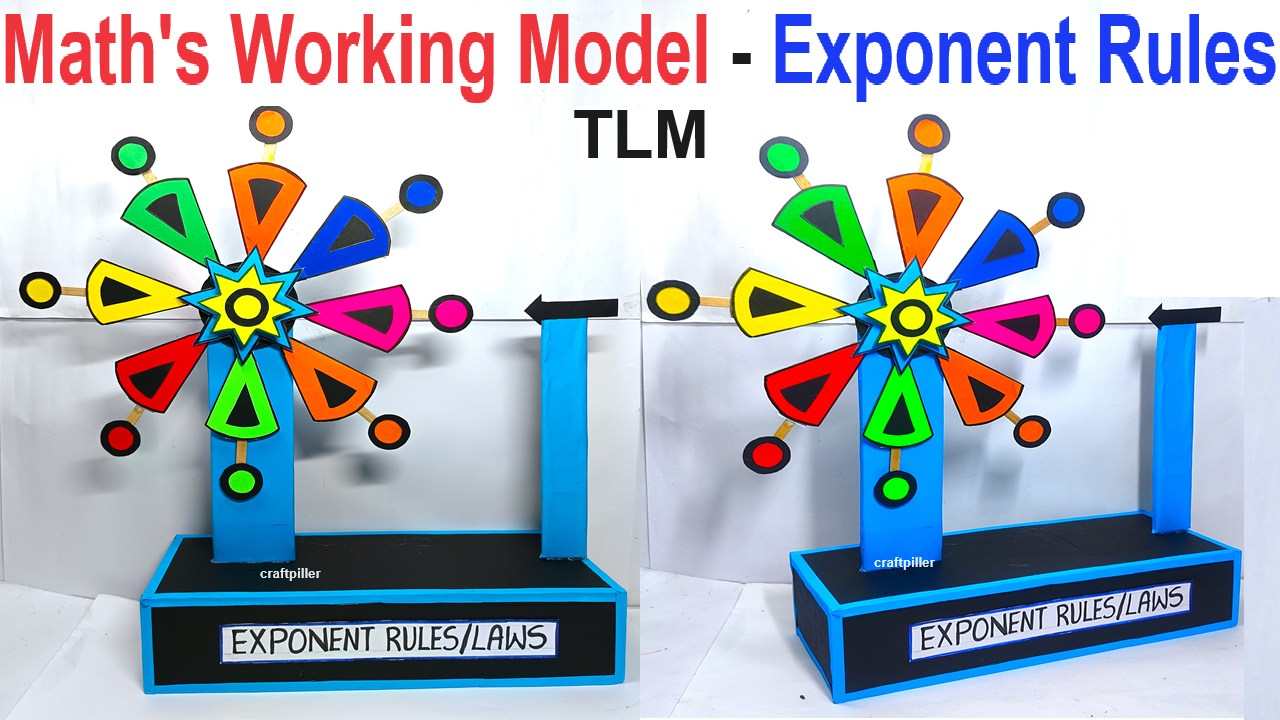
Creating a rotating model to demonstrate exponent rules in a circular pattern can be a great way to make the learning process interactive and engaging.
Here’s a step-by-step guide to help you create this working model using cardboard and colored paper:
Materials Needed:
- Cardboard
- Colored paper
- Scissors
- Glue
- Ruler
- Marker
- Brass fastener (paper fastener) or a small pivot mechanism
- Protractor (optional)
- String (optional for opening flaps)
- Tape
Steps by Step Video Instructions:
1. Prepare the Base and Rotating Circle:
- Cut the Cardboard Base: Cut a large circle from the cardboard (about 40 cm in diameter) to serve as the base.
- Cut the Rotating Circle: Cut a smaller circle from the cardboard (about 30 cm in diameter) to serve as the rotating part.
- Cover with Colored Paper: Cover both circles with colored paper to make them visually appealing.
2. Create the Sections:
- Divide the Base: Divide the larger circle into 8 equal sections using a ruler and marker. Each section will represent one exponent rule.
- Draw the Flaps: On the rotating circle, draw 8 segments similar to the base. These will be cut into flaps that can be opened to reveal the rules.
3. Cut the Flaps:
- Cut the Flaps: Carefully cut along the lines of each segment on the rotating circle, leaving one side attached so it can act as a hinge.
- Attach the Rotating Circle: Place the rotating circle on top of the base circle. Secure the center with a brass fastener or a small pivot mechanism, allowing the top circle to rotate.
4. Write the Rules, Definitions, and Examples:
- Exponent Rules: Write each exponent rule on the base circle within its designated section:
- Product of Powers Rule: am×an=am+na^m \times a^n = a^{m+n}am×an=am+n
- Quotient of Powers Rule: aman=am−n\frac{a^m}{a^n} = a^{m-n}anam=am−n
- Power of a Power Rule: (am)n=am×n(a^m)^n = a^{m \times n}(am)n=am×n
- Power of a Product Rule: (ab)n=an×bn(ab)^n = a^n \times b^n(ab)n=an×bn
- Power of a Quotient Rule: (ab)n=anbn\left(\frac{a}{b}\right)^n = \frac{a^n}{b^n}(ba)n=bnan
- Zero Exponent Rule: a0=1a^0 = 1a0=1 (where a≠0a \neq 0a=0)
- Negative Exponent Rule: a−n=1ana^{-n} = \frac{1}{a^n}a−n=an1
- Fractional Exponent Rule: amn=amna^{\frac{m}{n}} = \sqrt[n]{a^m}anm=nam
- Definitions and Examples: Write the definition and an example for each rule on a small piece of colored paper. Glue these inside each corresponding flap on the rotating circle. For example:
- Product of Powers Rule:
- Definition: When multiplying two powers with the same base, add the exponents.
- Example: 23×22=23+2=252^3 \times 2^2 = 2^{3+2} = 2^523×22=23+2=25
- Product of Powers Rule:
5. Assembly:
- Attach String (Optional): If you want to make the flaps easier to open, attach a small piece of string to the edge of each flap with tape.
- Secure the Flaps: Ensure each flap can open and close easily. The rotating circle should be able to turn to reveal each section one at a time.
- This interactive model will help in visually demonstrating the exponent rules, making it a fun and effective educational tool.