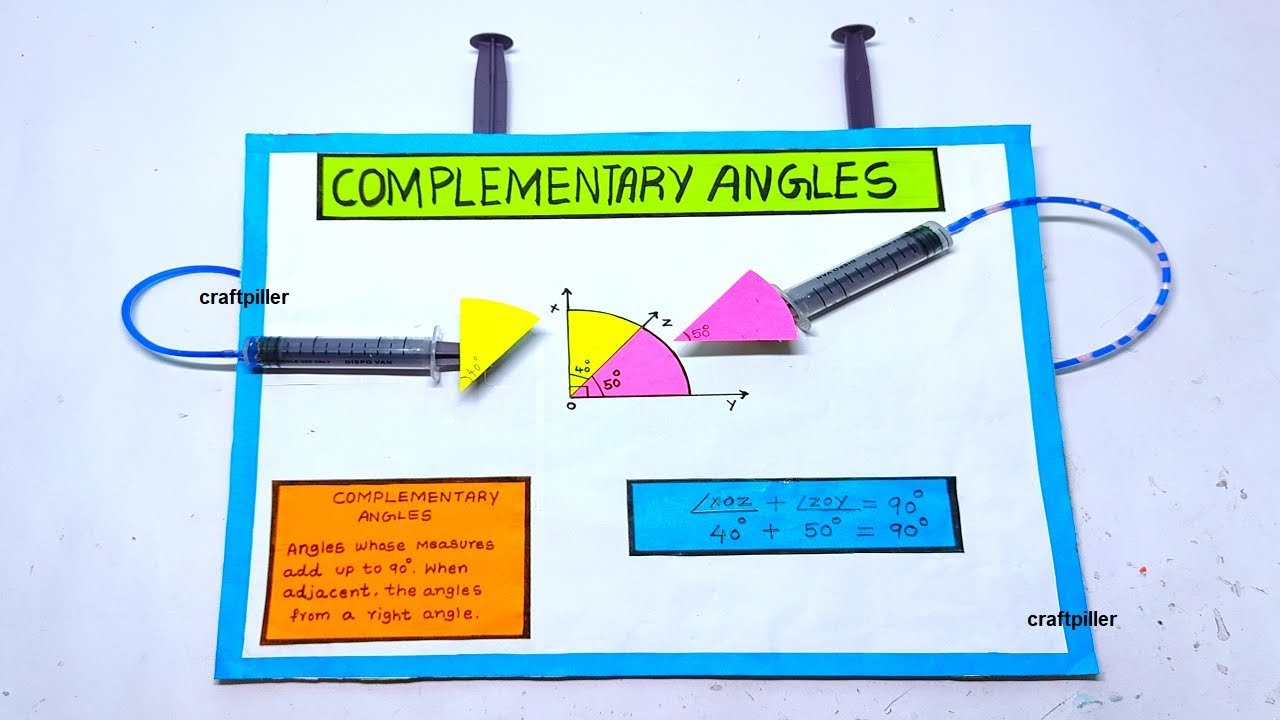
In this blog post we write about making of the complementary angles working model – maths tlm – diy – simple and easy – maths project
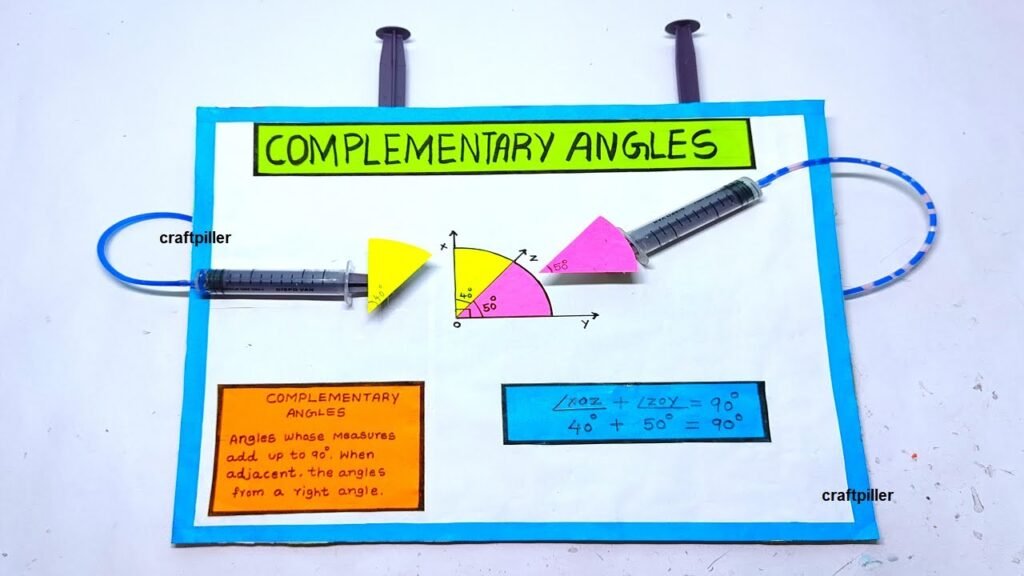
Complementary angles are two angles whose measures add up to 90 degrees. Complementary angles are often denoted with the symbol “∠” followed by a letter to represent the angle (such as ∠A and ∠B). For example, if ∠A measures 45 degrees, then ∠B must measure 45 degrees as well, since 45 + 45 = 90.
Complementary angles are always adjacent (sharing a common vertex and a common side), meaning that they are next to each other and form a straight line. Complementary angles can be acute (less than 90 degrees) or obtuse (more than 90 degrees) but cannot be right angles (90 degrees).
Complementary angles are an important concept in geometry and have many practical applications, such as in construction and engineering. For example, when building a wall or a structure, it is important to ensure that the angles are complementary in order to create a stable and strong foundation.
Remember, complementary angles add up to 90 degrees. If you know the measure of one angle, you can use this relationship to find the measure of the other angle.
#complementaryangles #workingmodel #mathstlm #mathsworkingmodel #scienceexhibition #sciencemodel #mathsproject