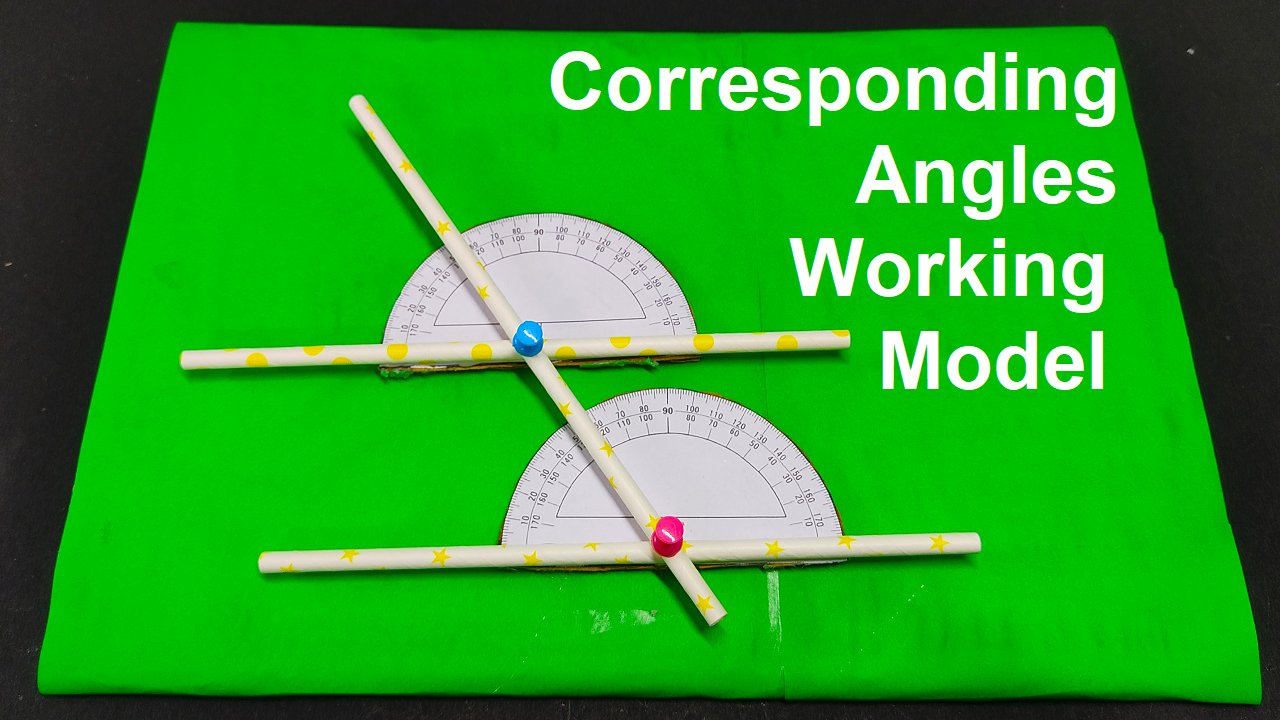
corresponding-angles-working-model-maths-exhibition-diy

how to make corresponding angles working model for math’s exhibition

by

Creating a working model to demonstrate corresponding angles using two protractors can be an effective way to illustrate this concept for a math exhibition.

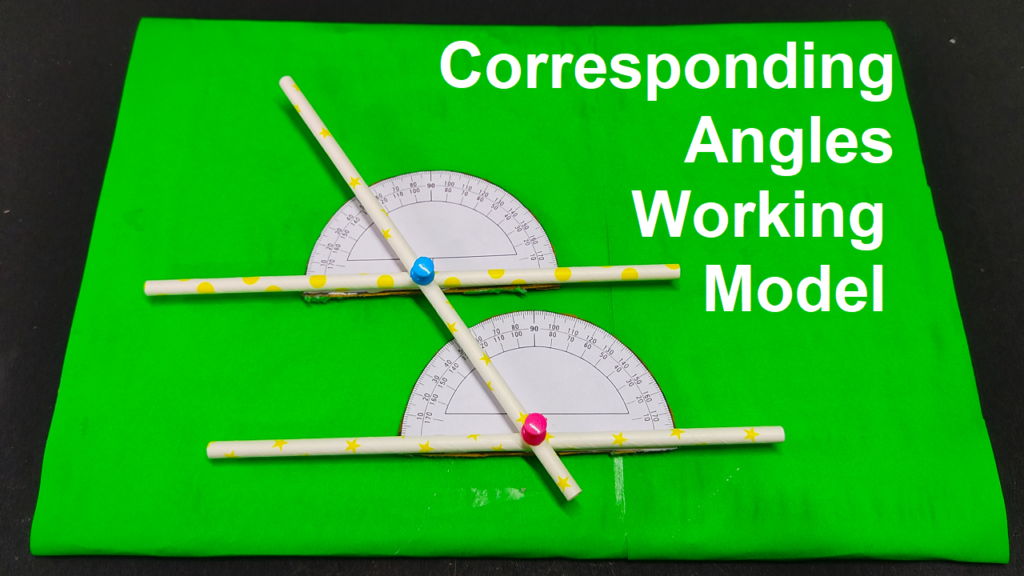
Here’s a simple DIY project using cardboard and two protractors:

Materials Needed:

  1. Two protractors
  2. Cardboard
  3. Ruler
  4. Pencil
  5. Compass
  6. Scissors
  7. Glue or tape
  8. Markers
  9. Wooden dowels or straws
  10. Small weights (e.g., small beads, paper clips)

Steps to Create the Corresponding Angles Working Model:

Step 1: Prepare the Base:

  • Cut a large piece of cardboard to serve as the base for your working model.

Step 2: Attach Protractors:

  • Glue or tape two protractors to the cardboard, ensuring that their centers align.

Step 3: Create Angle Templates:

  • Draw angle templates on colored paper or cardboard. These templates should represent corresponding angles in pairs.

Step 4: Label the Angles:

  • Label each angle template with the corresponding angle names (e.g., ∠A, ∠B, ∠C, ∠D).

Step 5: Attach Angles to Dowels:

  • Glue or tape each angle template onto wooden dowels or straws.

Step 6: Attach Dowels to the Base:

  • Attach the dowels or straws with the angle templates to the cardboard base. Place them in a way that allows for easy rotation.

Step 7: Add Weights (Optional):

  • Attach small weights (e.g., beads or paper clips) to the bottom of each angle template to help keep them in a vertical position.

Step 8: Display and Explain:

  • Set up your working model at the exhibition table.
  • Demonstrate corresponding angles by adjusting the angles on one protractor and observing the corresponding changes on the other protractor.

Step 9: Optional – Angle Facts:

  • Create small cards or labels to display interesting facts or properties about corresponding angles.

Explanation:

  • During your exhibition, explain the concept of corresponding angles and how they are related in geometric figures.
  • Allow visitors to interact with the model, adjusting the angles and observing the corresponding changes.

This working model provides a tangible representation of corresponding angles, allowing for an interactive and visual learning experience at a math exhibition. Visitors can physically manipulate the angles to see how corresponding angles are related, reinforcing their understanding of this geometric concept.

https://www.youtube.com/@diypandit

Leave a Comment