how to make Pythagorean theorem working model – Math’s Project – CraftPiller with explanation

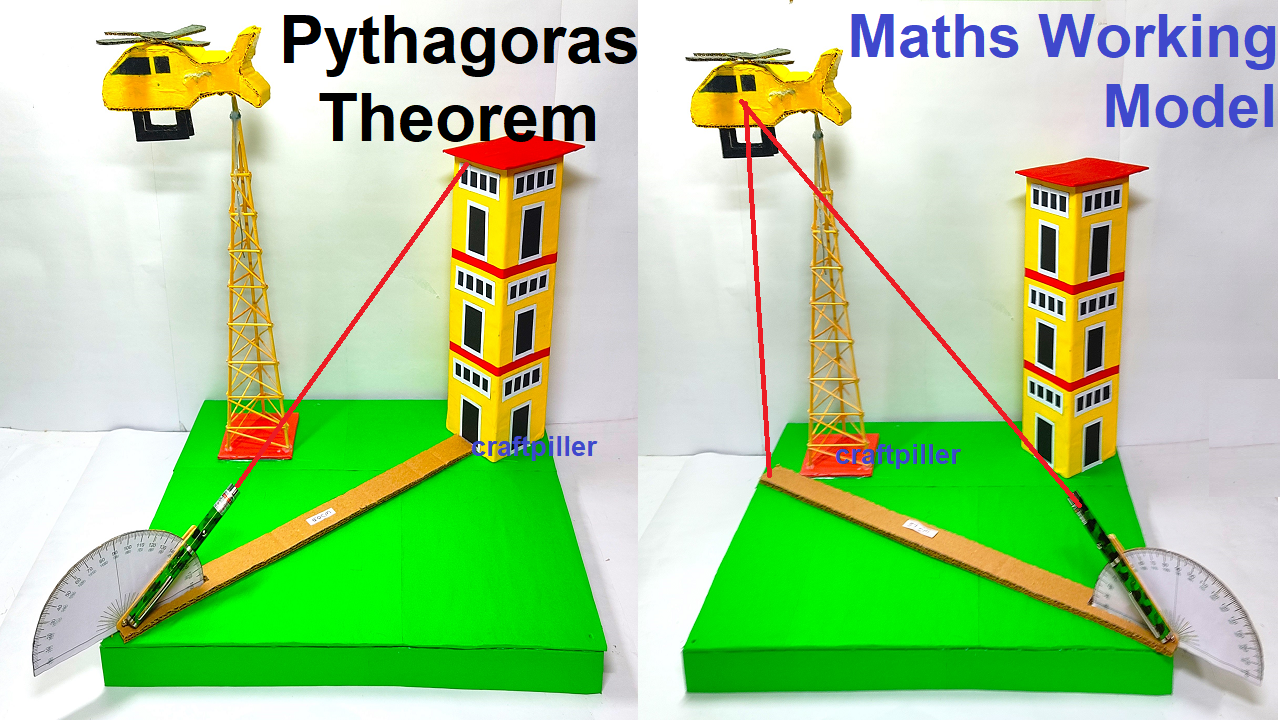
Creating a Pythagorean theorem working model using a helicopter and a building involves demonstrating the application of the theorem in a real-world scenario.

The Pythagorean theorem states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.

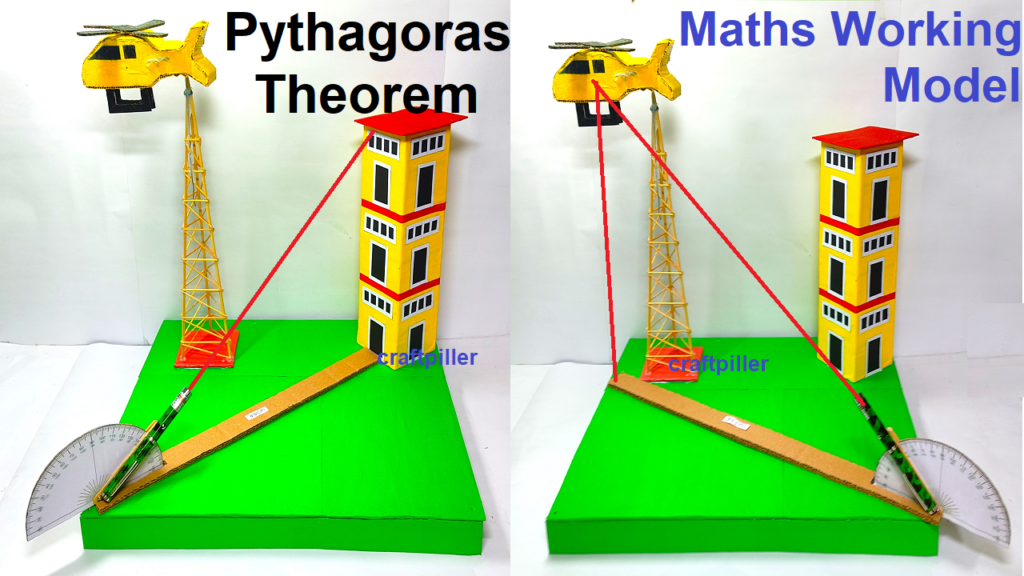
Here’s a simple working model using a cardboard helicopter and a building:

Materials Needed:

1. Cardboard helicopter
2. Small building or block structure
3. Measuring tape or ruler
4. Markers
5. Cardboard or poster board
6. Glue or tape
7. Scissors
8. Protractor (optional)

Pythagoras Theorem Working Model Construction:

1. Prepare the Building:
   • Use cardboard or poster board to create a small building or block structure. You can cut out rectangles to represent walls and a square for the base.
2. Set Up the Right Triangle:
   • Position the building so that it creates one leg of a right-angled triangle. This leg is the vertical side of the right triangle.
3. Position the Helicopter:
   • Place the toy helicopter at the base of the building, pointing away from the building.
4. Measure the Distance:
   • Use a measuring tape or ruler to measure the horizontal distance from the helicopter to the base of the building. This distance represents one leg of the right triangle.
5. Measure the Vertical Distance:
   • Measure the vertical distance from the helicopter to the top of the building. This distance represents the other leg of the right triangle.
6. Apply the Pythagorean Theorem:
   • Label the measured distances as ‘a’ and ‘b’ (the legs of the right triangle), and let ‘c’ be the hypotenuse (the distance from the helicopter to the top of the building).
   • Use the Pythagorean theorem: �2=�2+�2c2=a2+b2.
   • Square the lengths of ‘a’ and ‘b’, add them together, and take the square root to find ‘c’.

Demonstration:

1. Explanation:
   • Explain to your audience (students, peers, or viewers) that the Pythagorean theorem helps calculate distances in right-angled triangles.
2. Measurements:
   • Share the measured distances ‘a’ and ‘b’ with your audience and discuss how these represent the legs of the right triangle.
3. Calculation:
   • Perform the calculation using the Pythagorean theorem to find the distance ‘c.’
4. Verification:
   • Use the toy helicopter to physically measure the calculated distance ‘c’ and verify that it matches the actual measurement.

https://www.youtube.com/@craftpiller