Introduction to Newtonian Rotational Motion and Moment of Inertia:
Understanding rotational motion and moment of inertia is essential in physics, particularly when dealing with objects that rotate.
Sir Isaac Newton’s laws of motion, which describe the relationship between the motion of an object and the forces acting on it, are extended to cover rotational motion.
Additionally, the concept of moment of inertia plays a crucial role in understanding how objects resist changes in their rotational motion.
Newton’s Laws Applied to Rotational Motion:
- First Law of Rotational Motion (Newton’s First Law for Rotation):
- Newton’s first law states that an object at rest will stay at rest, and an object in motion will stay in motion unless acted upon by an external torque. In rotational terms, this law implies that an object will keep rotating with a constant angular velocity unless an external torque is applied.
- Second Law of Rotational Motion (Newton’s Second Law for Rotation):
- Newton’s second law for rotation relates the net torque applied to an object to its moment of inertia and angular acceleration. The equation is τ = Iα, where τ is the torque, I is the moment of inertia, and α is the angular acceleration. This law is analogous to F = ma in linear motion.
- Third Law of Rotational Motion (Newton’s Third Law for Rotation):
- Newton’s third law for rotation is similar to the linear counterpart and states that for every action torque, there is an equal and opposite reaction torque. This law highlights the conservation of angular momentum.
Moment of Inertia:
- Definition:
- The moment of inertia (I) is a measure of an object’s resistance to changes in its rotational motion. It depends not only on the mass of the object but also on how that mass is distributed relative to the axis of rotation.
- Calculation:
- The moment of inertia is calculated using the formula I = Σmiri², where Σmiri² is the sum of the products of each particle’s mass (mi) and its square of the perpendicular distance (ri) from the axis of rotation.
- Effect on Rotation:
- Objects with larger moment of inertia require more torque to produce the same angular acceleration. For example, it’s easier to spin a slender rod about its axis than a solid disk of the same mass.
- Parallel Axis Theorem:
- The parallel axis theorem allows one to calculate the moment of inertia for an axis parallel to an axis through the center of mass. It is given by I_parallel = I_cm + Md², where I_parallel is the moment of inertia for the parallel axis, I_cm is the moment of inertia for the axis through the center of mass, M is the total mass of the object, and d is the perpendicular distance between the two axes.
Applications:
- Gyroscopes:
- Gyroscopes utilize the principles of rotational motion and moment of inertia to maintain orientation and stability in navigation systems.
- Spinning Objects:
- Understanding rotational motion is crucial in sports such as figure skating, gymnastics, and diving, where athletes manipulate their bodies’ rotations.
- Rotational Machinery:
- In engineering, the design of rotating machinery, such as flywheels and turbines, involves considerations of rotational motion and moment of inertia for efficient and stable operation.
working model to demonstrate Newton’s second law of motion
Creating a working model to demonstrate Newton’s second law of motion with rotational motion and moment of inertia can be a fascinating project.
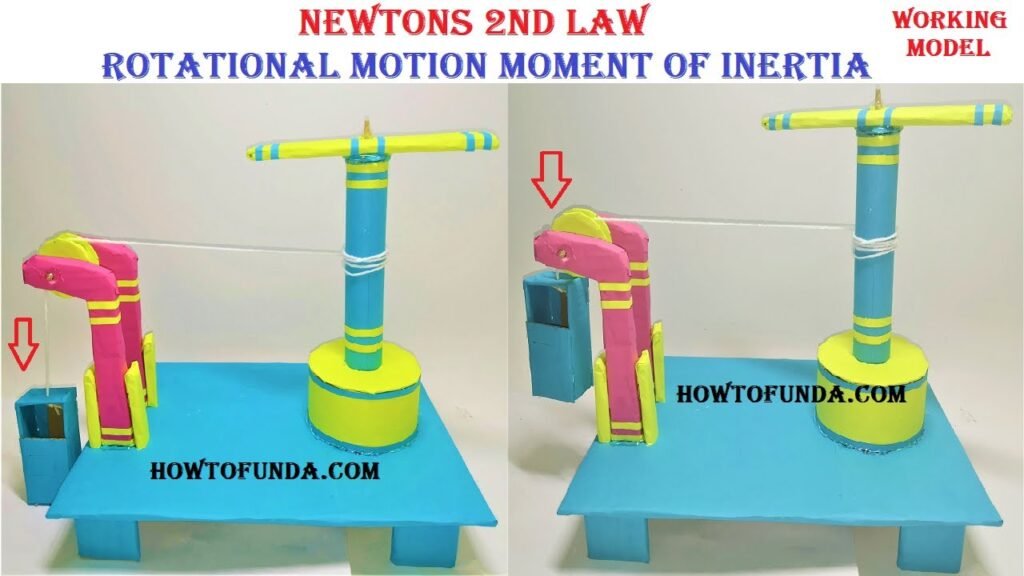
Here’s a step-by-step guide using a rotating platform:
Materials Needed:
- A rotating platform (can be a lazy Susan or any turntable)
- Small objects with known masses (e.g., small weights, coins)
- String
- Tape
- Ruler
- Stopwatch or timer
- Hole punch (optional)
- Cardboard or lightweight material
- Marker
- Small pulley (optional)
- Small weights or counterweights (optional)
Step by Step Video Procedure:
1. Set Up the Rotating Platform:
- Use a lazy Susan or any other rotating platform as the base for your model. Ensure it can rotate freely.
2. Prepare the Objects:
- Attach small objects of known masses to strings. You can use tape to secure the strings to the objects. Ensure that the strings are long enough to reach the floor when the objects are on the rotating platform.
3. Create the Moment of Inertia System:
- Attach the strings to the rotating platform. You can tape them near the edge so that the objects can hang freely.
4. Measure the Radius:
- Measure the distance from the center of the rotating platform to the point where the strings are attached. This distance is the radius (r) in the moment of inertia equation.
5. Determine the Angular Acceleration:
- Rotate the platform and release the objects. Time how long it takes for the objects to reach the bottom. Use the measured time to calculate the angular acceleration (α) using the formula α = 2π / T, where T is the period of rotation.
6. Calculate Moment of Inertia:
- Use the formula for moment of inertia (I = m * r^2) to calculate the moment of inertia for each object. m is the mass of the object, and r is the radius.
7. Experiment with Different Masses:
- Repeat the process with objects of different masses. Observe how changes in mass affect the angular acceleration and moment of inertia.
8. Discuss Newton’s Second Law:
- Explain that according to Newton’s second law for rotational motion, the torque (τ) applied to an object is equal to the moment of inertia (I) multiplied by the angular acceleration (α): τ = I * α.
9. Discuss Inertia:
- Discuss how moment of inertia is a measure of an object’s resistance to changes in its rotation. Increasing the mass or redistributing the mass away from the axis of rotation increases the moment of inertia.
10. Optional: Pulley System and Counterweights: – For an added challenge, you can create a pulley system and counterweights to experiment with changing the moment of inertia dynamically.
11. Record Observations: – Record your observations and note how changes in mass and distribution affect the rotational motion.
Conclusion:
The extension of Newton’s laws to rotational motion, along with the concept of moment of inertia, provides a comprehensive framework for understanding how objects rotate and respond to applied torques.
These principles have broad applications in physics, engineering, and various real-world scenarios involving rotational dynamics.

