Here are 25 math’s working project for class 10 students
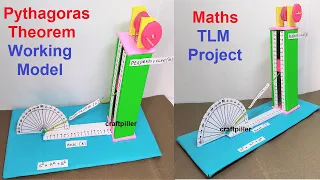
1) Pythagorean Theorem Working Model
The Pythagorean Theorem is a mathematical rule that helps us find the length of one side of a right-angled triangle when we know the lengths of the other two sides.
A working model to demonstrate the Pythagorean Theorem could be a physical representation of a right-angled triangle along with squares on each side. Here’s how you can explain it in layman terms:
- The Right-Angled Triangle: Imagine a triangle with one corner forming a perfect right angle, like the corner of a piece of paper. This is a right-angled triangle.
- The Short Side (A): One side of the triangle is shorter, and we’ll call it ‘A’. It’s like the width of a rectangular plot of land.
- The Other Short Side (B): Another side is also short, and we’ll call it ‘B’. It’s like the length of the same rectangular plot of land.
- The Long Side (C): The third side, opposite the right angle, is longer and we’ll call it ‘C’. It’s like the diagonal of the rectangular plot.
- The Squares: Now, we’re going to make squares on each of these sides. For side ‘A’, make a square with ‘A’ on each side. Do the same for side ‘B’ and side ‘C’.
- The Interesting Discovery: Here’s the magic part! When you compare the area of the square on side ‘A’ plus the area of the square on side ‘B’, it’s exactly the same as the area of the square on side ‘C’!
- The Rule: This magical equality is the Pythagorean Theorem. It says that in any right-angled triangle, the square of the length of the hypotenuse (the longest side, ‘C’) is equal to the sum of the squares of the other two sides (‘A’ and ‘B’).
So, in simple words, the Pythagorean Theorem is like a secret trick that helps us figure out the lengths of sides in a right-angled triangle. It’s as if the squares on the shorter sides add up perfectly to the square on the longer side! That’s why it’s so useful in geometry and real-life situations where triangles are involved.
2) Trigonometric Ratios Working Model
Trigonometric ratios are a way of understanding the relationships between the sides of a right-angled triangle.
Let’s explain this using a simple working model:
- The Right-Angled Triangle: Imagine a triangle with one corner forming a perfect right angle, just like the corner of a piece of paper.
- The Short Side (Adjacent): One side of the triangle is shorter, and we’ll call it the “adjacent” side. It’s like the width of a rectangular plot of land.
- The Tall Side (Opposite): The side opposite to the right angle is taller, and we’ll call it the “opposite” side. It’s like the length of the same rectangular plot of land.
- The Hypotenuse (Diagonal): The longest side, opposite the right angle, is called the “hypotenuse.” It’s like the diagonal of the rectangular plot.
- Using Trigonometric Ratios: Now, let’s say we’re interested in finding the relationship between these sides. There are three main trigonometric ratios we use:
- Sine (sinθ): This is like a magic number that tells us the ratio of the “opposite” side to the “hypotenuse.” It’s like finding out what part of the rectangular plot’s length is taken up by its diagonal.
- Cosine (cosθ): This is another magic number that tells us the ratio of the “adjacent” side to the “hypotenuse.” It’s like finding out what part of the rectangular plot’s width is taken up by its diagonal.
- Tangent (tanθ): This one tells us the ratio of the “opposite” side to the “adjacent” side. It’s like finding out how much taller the plot is compared to how wide it is.
- Practical Example: Imagine you’re looking at a flagpole. If you know how far you are from the flagpole and the angle your line of sight makes with the ground, you can use these trigonometric ratios to figure out how tall the flagpole is.
So, in simple words, trigonometric ratios are like special tools that help us understand the relationships between the sides of a right-angled triangle. They’re really handy for things like measuring heights, distances, and angles in real-life situations!
3.Probability Working Model
Probability is a branch of mathematics that deals with the likelihood or chance of events occurring.
It provides a way to quantify uncertainty and make predictions based on available information.
Probability is used extensively in various fields such as statistics, economics, physics, and more.
4)Exponents and Powers Working Model
Exponents and powers are fundamental concepts in mathematics that involve raising a number to a certain power or exponent.
They play a crucial role in representing repeated multiplication and are widely used in various fields, including algebra, calculus, and science.
5)Mensuration Working Model
Mensuration is a branch of mathematics that deals with the measurement of geometric figures and their related parameters, such as length, area, volume, and surface area.
It is an essential part of geometry and has widespread applications in various fields, including engineering, architecture, physics, and more.
6)Coordinate Geometry Working Model
Coordinate geometry, also known as analytical geometry, is a branch of mathematics that combines elements of algebra and geometry.
It involves using algebraic techniques to study geometric properties and solve geometric problems.
7)Algebraic Identities Working Model
Algebraic identities are essential mathematical rules that express the relationship between different algebraic expressions.
They provide a framework for simplifying and manipulating equations in algebra.
These identities serve as shortcuts or patterns that help us perform calculations more efficiently. They play a crucial role in solving equations, factoring expressions, and making complex algebraic operations more manageable.
8)Circles Working Models
A circle is a fundamental geometric shape that has played a significant role in mathematics, engineering, and various fields of science.
It is defined as the set of all points in a plane that are equidistant (at the same distance) from a fixed point called the center.
9)Real Numbers Working Model
Real numbers are a fundamental concept in mathematics that encompasses a vast set of numbers used to represent quantities in various contexts.
They include both rational numbers (fractions) and irrational numbers (non-repeating, non-terminating decimals).
10)Linear Equations Working Models
Linear equations are fundamental mathematical expressions that describe a straight-line relationship between two variables. They play a crucial role in various fields such as physics, engineering, economics, and more.

